Devoirs Corrigés Maths N°2 S1 1AC
Modèle N°1
Exercice 1:$(0,5+0,5+0,5+0,5+1+1+1=5 pts)$
1) Calculer :
$A=(-8)+(+6)$
$\mathbf{B}=(-5,7)+(-3,8)$
$\mathbf{C}=24 -(\mathbf{- 1 3})$
$\mathbf{D}=34,7-50$
$\mathbf{E}=(-7)-12+(-5)$
$\mathrm{F}=\mathbf{9}-\mathbf{5 , 3}-(-5)-3,7$
$\mathbf{G}=-3+14+7-8-11$
Exercice 2:$(2 pts)$
Supprimer les parenthèses et le crochet et calculer l’expression suivante:
$H = 18 – (17 + 4 – 6 ) – [13 – (4 – 18 ) ]$
Exercice 3:$(1,5+1,5=3 pts)$
$a$ et $b$ deux nombres relatifs tels que : $a+b=-11 \quad$
Calculer :
$M=(\mathbf{a}-\mathbf{9}+\mathbf{4 5})-(5-b-45)$
$N=45,3-[7+b-(-30)]-(a+15,3)$
Exercice 4:$(0,5+0,75+0,75=2pts)$
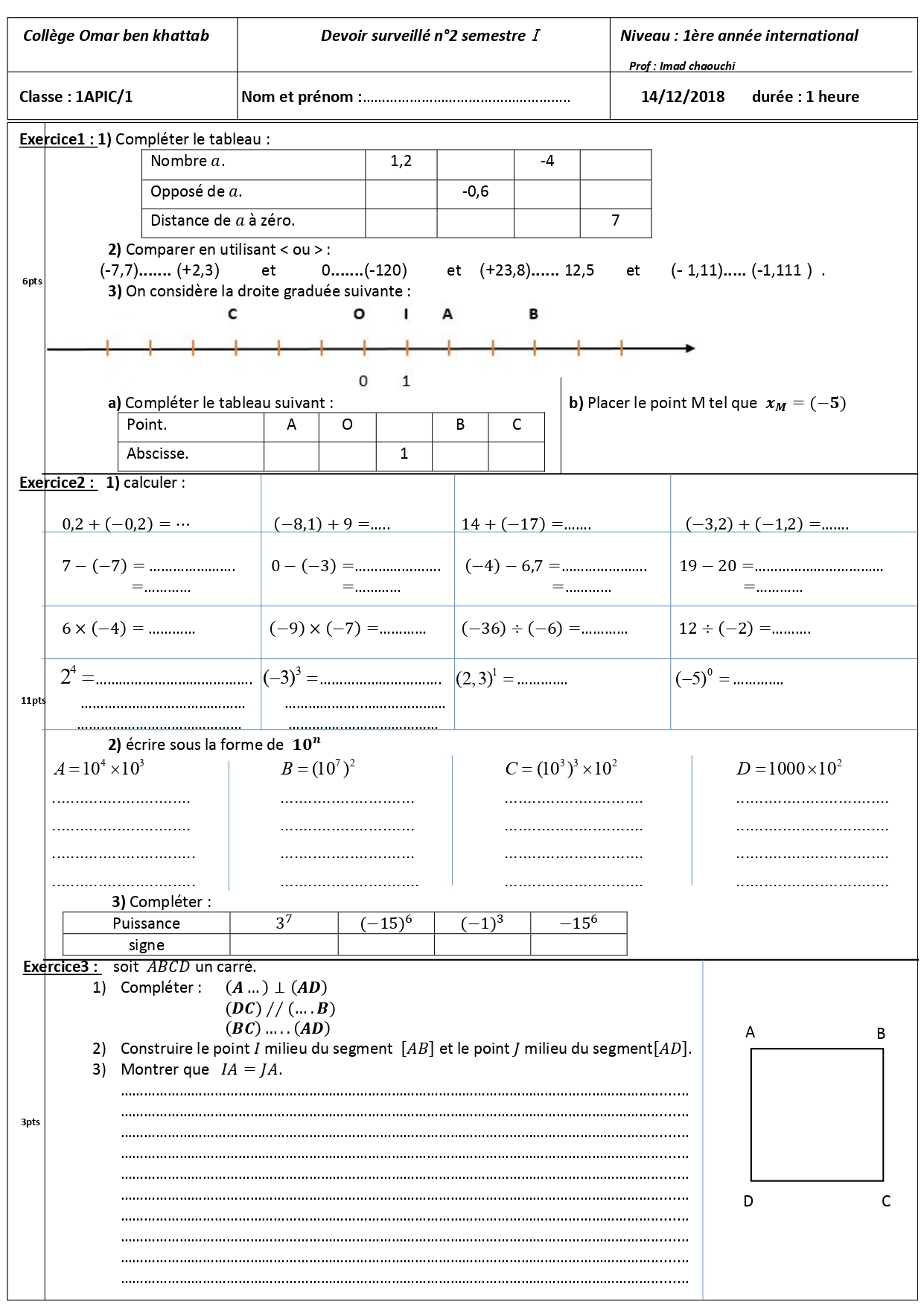
Observer la figure puis compléter.
• Les deux angles $\mathbf{T} \hat{S} O$ et $………$ sont opposés par le sommet.
• Les deux angles $\mathrm{T} \hat{S} \mathrm{D}$ et $………$ sont supplémentaires.
• Les deux angles $………$ et $………$ sont complémentaires.
Exercice 5:$(1 +1+1+1=4 pts)$
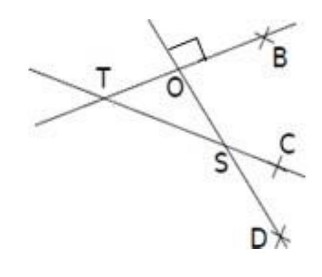
On considère la figure ci-dessous :
Calculer en justifiant votre réponse la mesure de chacun des angles suivants:
a- $C \widehat{A} \mathbf{E}$
b- $A \widehat{D} \mathbf{B}$
c- $E \widehat{A} \mathbf{D}$
d- $C \widehat{A} \mathbf{D}$
Exercice 6:$(1 +1+0,5+0,5+1=4 pts)$
a) Construire le triangle $MNP$ isocèle en $P$ tel que $\mathrm{MN}=4 \mathrm{~cm}$; $\mathbf{N} \widehat{\mathbf{M}} \mathbf{P}=70^{\circ}$
b) Calculer la mesure d’angle $\mathbf{M} \widehat{\boldsymbol{P}} \boldsymbol{N}$
c) Placer le point $H$ la projection orthogonale de $M$ sur la droite $(PN)$
d) Déterminer la nature du triangle $MNH$ ? justifier votre réponse
e) Calculer en justifiant votre réponse la mesure d’angle $\mathrm{H} \widehat{\boldsymbol{M}} \boldsymbol{N}$
$A=(-8)+(+6) $
$A=-8+6 $
$A=-2$
$\mathrm{B}=(-\mathbf{5 , 7})+(-3,8) $
$ \mathrm{B}=-5,7-3,8 $
$\mathrm{~B}=-9,5$
$ \mathrm{C}=24-(-13 $
$ \mathrm{C}=24+13 $
$ \mathrm{C}=37$
$ \mathbf{D}=\mathbf{3 4 , 7}-\mathbf{5 0}$
$\mathbf{D}=-(50-34,7) $
$\mathbf{D}=-15,3$
$\mathbf{E}=(-7)-12+(-5) $
$ E=-7-12-5 $
$ E=-(7+12+5) $
$E=-24$
$ \mathrm{F}=\mathbf{9}-\mathbf{5 , 3}-(-5)-3,7 $
$ \mathrm{F}=9-\mathbf{5 , 3} +5-3,7$
$ \mathrm{F}=9+\mathbf{5}-\mathbf{5 , 3}-3,7 $
$ \mathrm{F}=\mathbf{1 4}-\mathbf{9}$
$ \mathrm{F}=5$
$ \mathbf{G}=-3+14+7-8-11 $
$ \mathbf{G}=+14+7-3-8-11 $
$ \mathbf{G}=+21-22 $
$\mathbf{G}=-(22-21)$
$\mathbf{G}=-1$
$\mathrm{H} = 18 – (17 + 4 – 6 ) – [13 – (4 – 18 ) ]$
$\mathrm{H}=18-17-4+6-13+(4-18)$
$\mathrm{H}=1 8-1 7-4 + 6 -1 3+4 – 18$
$\mathrm{H}=1 8+6 + 4-1 7 – 4 – 1 3 – 1 8$
$\mathbf{H}=2 8-5 2$
$\mathrm{H}=-(52-28)=-24$
• $M=(\mathbf{a}-\mathbf{9}+\mathbf{4 5})-(5-b-45)$
$M=a-9+45-5+b+45$
$M=a+b-9-5+45+45$
$M=-11-9-5+45+45$
$M=-25+90$
$M=65$
• $N=45,3-[7+b-(-30)]-(a+15,3)$
$\mathrm{N}=45,3 – 7 – b + (-30) – a – 15,3$
$\mathrm{N}=\mathbf{4 5 , 3} – 7 – b – 30 – a – 15,3$
$\mathrm{N}=\mathbf{4 5 , 3} – a – b- 7 – \mathbf{3 0} – 15,3$
$\mathrm{N}=45,3-(\mathrm{a}+\mathrm{b})-7 – \mathbf{3 0} – 15,3$
$\mathrm{N}=45,3-(-11)-7-30-15,3$
$\mathrm{N}=\mathbf{4 5 , 3}+\mathbf{1 1 – 7 – 3 0}- 15,3$
$\mathbf{N}=\mathbf{5 6 , 3} – 52,3$
$\mathrm{N}=4$
• Les deux angles $\mathbf{T} \hat{S} O$ et $\mathbf{D} \hat{S} \mathbf{C}$ sont opposés par le sommet.
• Les deux angles $\mathrm{T} \hat{S} \mathrm{D}$ et $\mathbf{D} \hat{\boldsymbol{S}} \mathbf{C}$ sont supplémentaires.
$\operatorname{Car}\left(\mathbf{T} \hat{S} \mathbf{D}+\mathbf{D} \hat{S} \mathbf{C}=\mathbf{1 8 0}{ }^{\circ}\right)$
• Les deux angles $\mathbf{S} \widehat{T} \mathbf{O}$ et $\mathbf{O} \widehat{S} \mathbf{T}$ sont complémentaires.
$\operatorname{Car}(\mathbf{S} \widehat{T} \mathbf{O}+\mathbf{O} \widehat{\mathbf{S}} \mathbf{T}=\mathbf{9 0}^{\circ} \mathbf{} \mathbf{)}$
a- $C \widehat{A} \mathbf{E}$
Puisque le triangle $A C E$ est rectangle en $E$ et isocèle alors $\mathrm{A} \widehat{E} C=90^{\circ}$ et $C \widehat{A} E=A \widehat{C} E$
Donc: $C\widehat{A} E=\frac{(180^{\circ}-90^{\circ})}{2}$
$\mathrm{C} \widehat{A} \mathrm{E}=45^{\circ}$
b- $A \widehat{D} \mathbf{B}$
Puisque le triangle ADB est isocèle alors :
$\mathbf{D} \widehat{A} B=\mathbf{D} \widehat{B} \mathbf{A}=31^{\circ}$
Donc: $\mathbf{A} \widehat{D} B=180^{\circ}-\left(31^{\circ}+31^{\circ}\right)$
$\mathrm{A} \widehat{D} B=118^{\circ}$
c- $E \widehat{A} \mathbf{D}$
Dans le triangle $AED$ on a : $\mathbf{A} \widehat{E }D=90^{\circ}$ et $A \widehat{D}E=180^{\circ}-118^{\circ}=62^{\circ}$
Donc : $ \mathbf{E} \widehat{A} \mathrm{D}=180^{\circ}-\left(90^{\circ}+62^{\circ}\right)=180^{\circ}-152^{\circ}$
$\mathbf{E} \widehat{A} \mathrm{D} =28^{\circ}$
d- $C \widehat{A} \mathbf{D}$
$C\widehat{A} D=C\widehat{A} E+\mathbf{E} \widehat{\mathbf{A}} \mathbf{D}$
$C\widehat{A} D =45^{\circ}+28^{\circ}$
$C\widehat{A} D=73^{\circ}$
a)
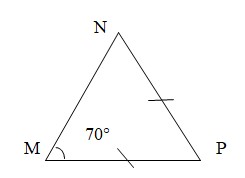
b) Le triangle MNP isocèle en P , Alors $\mathbf{M} \widehat{N} \mathbf{P}=\mathbf{N} \widehat{M} \mathbf{P}=70^{\circ}$
Donc : $\mathbf{M} \widehat{\boldsymbol{P}} \boldsymbol{N}=\mathbf{1 8 0 ^ { \circ }}-\left(\mathbf{7 0}{ }^{\circ}+\mathbf{7 0}{ }^{\circ}\right)=\mathbf{4 0}{ }^{\circ}$
c)
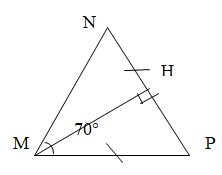
d) Le triangle $MNH$ est rectangle en $H$ car $H$ est la projection orthogonale de $M$ sur la droite $(PN)$.
e) $H \widehat{M} N=180^{\circ}-(M \widehat{H} N+H \widehat{N} M)$
$H \widehat{M} N=180^{\circ}-\left(90^{\circ}+70^{\circ}\right)$
$H \widehat{M} N=180^{\circ}-\left(160^{\circ}\right)$
$H \widehat{M} N=20^{\circ}$
Modèle N°2
Exercice 1:$(14 pts)$
1) Calculer les opérations suivantes: $(1 \times 6=6 pts)$
$(-4,3)+(-5) \quad ; \quad 17+(-7) \quad ; \quad(-8,5)+(-15)$
$5-(-8) \quad ; \quad 17,8-12,8 \quad ; \quad(-9)-(-5,4)$
2) Comparer les nombres relatifs suivants : $(0,5 \times 6=3 pts)$
$(-4,3) \ldots . .(-5)$
$15 ….. (-7) $
$(-8,5) \ldots . .(-15)$
$0 ….. (-8)$
$-17,8 …. .17,8$
$(-9)…. . (-5,4)$
3) Range dans l’ordre croissant : $(1 pt)$
$-5 \quad ; \quad -15 \quad ; \quad 0 \quad ; \quad -2,5 \quad ; \quad 2 \quad ; \quad 3,5 \quad ; \quad -13,51$
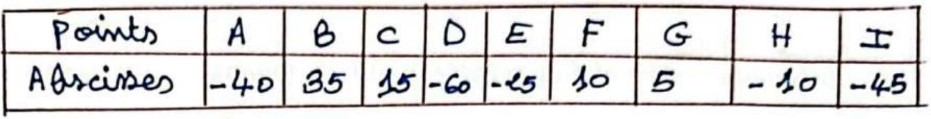
4) On considéré les abscisses des points suivants : $(1+2+1=4pts)$
$E(-3) \quad ; \quad F(2,5) \quad ; \quad G(-2) \quad ; \quad K(1,5)$
a) Place les points sur une droite graduée.
b) Calculer les distances $EF$ et $FG$.
c) Déterminer l’abscisse de $M$ sachant que $M$ est le milieu de $[EG]$.
Exercice 2:$(1,5+1+0,5+1+1+1=6pts)$
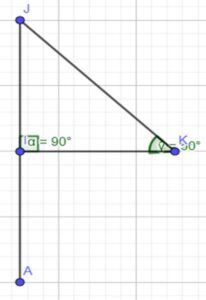
$IJK$ triangle rectangle en $I$ tel que : $\mathrm{IJ}=5 \mathrm{~cm}$ et $I \hat{\jmath} K=50^{\circ}$
1) Déterminer la valeur de $I \hat{J} K$ ? Justifier
2) Construire le triangle $IJK$.
3) Construire le point $A$ de demi-droite $[AJ)$ tel que $I$ est le milieu de $[AJ]$.
4) Que représente la droite $(IK)$ par rapport au segment $[AJ]$ ? Justifier
5) Donner la nature de triangle $AKJ$ ? Justifier
6) Déterminer la valeur de $\widehat{K A} J$ ? Justifier
1) Calculer les opérations suivantes: $(1 \times 6=6 pts)$
$(-4,3)+(-5)=-8,3$
$ 17+(-7)=17-7=10$
$(-8,5)+(-15)=-23,5$
$ 5-(-8)=5+8=13$
$17,8-12,8=5 $
$(-9)-(-5,4)=-9+5,4=-(9-5,4)=-3,6$
2) Comparer les nombres relatifs suivants : $(0,5 \times 6=3 pts)$
$(-4,3) > (-5)$
$15 > (-7) $
$(-8,5) > (-15)$
$0 > (-8)$
$-17,8 < 17,8$
$(-9) < (-5,4)$
3) Range dans l’ordre croissant : $(1 pts)$
$-15<-13,51<-5<-2,5<0<2<3,5$
4) On considéré les abscisses des points suivants : $(1+2+1=4pts)$
$E(-3) \quad ; \quad F(2,5) \quad ; \quad G(-2) \quad ; \quad K(1,5)$
a) Place les points sur une droite graduée.
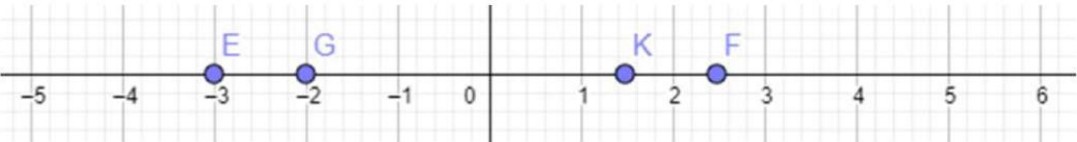
b) Calculer les distances $EF$ et $FG$.
– Pour calculer $EF$ il faut comparer l’abscisse de $E$ avec l’abscisse de $F$ :
Nous avons : $2,5>-3$
Alors : $\mathrm{EF}=2,5-(-3)=2,5+3=5,5$
– Pour calculer $FG$ il faut comparer l’abscisse de $\mathbf{F}$ avec l’abscisse de $G$ :
Nous avons: $2,5>-2$
Alors : $\mathrm{FG}=2,5-(-2)=2,5+2=4,5$
c) Déterminer l’abscisse de $M$ sachant que $M$ est le milieu de $[EG]$.
$M$ est le milieu de [EG], c’est-à-dire : $x_{M}=-2,5$
1) Déterminer la valeur de $I \hat{J} K$ ? Justifier
La somme des angles de ce triangle égale à $180^{\circ}$ :
$I \hat{J} K+I \widehat{K} J+J \hat{I} K=180^{\circ}$
Alors: $I \hat{J} K=180^{\circ}-(I \widehat{K} J+J \hat{I} K)$
$I \hat{J} K=180^{\circ}-\left(50^{\circ}+90^{\circ}\right)$
Donc: $I \hat{J} K=40^{\circ}$
2) Construire le triangle $IJK$.
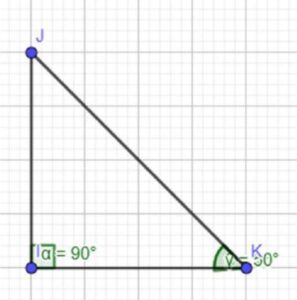
3) Construire le point $A$ de demi-droite $[AJ)$ tel que $I$ est le milieu de $[AJ]$.
4) Que représente la droite $(IK)$ par rapport au segment $[AJ]$ ? Justifier
La droite $(IK)$ est la médiatrice du segment $[AJ]$, car $I$ est le milieu du segment $[AJ]$ et la droite $(IK)$ perpendiculaire à la droite $(AJ)$.
5) Donner la nature de triangle $AKJ$ ? Justifier
Puisque la droite $(IK)$ est la médiatrice du segment $[AJ]$, alors $[JK] = [AK]$
Donc le triangle $AKJ$ est isocèle.
6) Déterminer la valeur de $\widehat{K A} J$ ? Justifier
Le triangle $AKJ $ est isocèle.
Alors : $K \widehat{A} J=A \widehat{J} K=I \widehat{J} K=40^{\circ}$
Modèle N°3
Exercice 1:$(14 pts)$
1) Compléter par le signe ” < ” ou ” >” :: $(1,5 pts)$
$+2,67 … 5,01$
$ -70,7 … – 1,5$
$+13 …- 23$
2) Ranger les nombres suivants dans l’ordre croissant: : $(1 pt)$
$-7,12 \quad ; \quad -19 \quad ; \quad 11 \quad ; \quad +3,1 \quad ; \quad 1 \quad ; \quad 0 \quad ; \quad -9,9 \quad ; \quad -11,01 $
3) On Considère la droite graduée ci-dessous : $(1+1=2 pt)$
a. Placer sur la droite les points .$A (-5)$, $\mathrm{B}(+3), \boldsymbol{C}(-2,5)$ et $D(+5)$ :
b. Calculer les distances $AB$ et $CD$ .
4) Calculer les expressions suivantes en écrivant les étapes intermédiaires : $(0,5+0,5+0,5+0,5+2=4pts)$
$(-3)+7 $
$-90 \div 45$
$(+2)-(-18) $
$(-18) \times(+0,5)$
$(-6)+(-6) \times(+2)+(-36) \div(-3)+3 \times(+2)$
5) Sachant que $a+b=-3$ et $a \times b=4$, calculer:$(0,75+0,75 =1,5 pt)$
• $1+a+(-5)+b+(+7) $
• $ a \times(-1) \times b-(-4)$
6) Supprimer les parenthèses puis effectuer les calculs : $(1 pt)$
$\mathrm{D}=-(-12-(+3-7))+(-12+4)$
Exercice 2:$(1+1+1=3 pts)$
Peut -on construire le triangle ABC dans les cas suivants? justifier ta réponse.
• $A B=7 \mathrm{~cm}, A \hat{B} C=95^{\circ}, B A \hat{ C}=87^{\circ}$
• $A B=3 \mathrm{dm} ; A C=4 \mathrm{~cm} ; B C=6 \mathrm{~cm}$
• $A B=5 \mathrm{~cm} ; A C=5 \mathrm{~cm} ; B \hat{A} C=60^{\circ} ; A \widehat{B} C=90^{\circ}$
Exercice 3:$(5 pts)$
1) Soit $A B C$ un triangle isocèle en $A$ tel que: $\mathrm{BC}=4 \mathrm{~cm}$ et $A \widehat{\mathrm{B}}C=\mathbf{5 0 ^ { \circ }}$$(1+1=2 pts)$
a) Construire la figure
b) Calculer la mesure de $B \widehat{A} C$ en justifiant
2) Observer la figure ci-dessous. tel que $E \widehat{O} L=90^{\circ}$ et $N \widehat{K}M=\mathbf{6 0} ^{\circ}$ et $F \widehat{O} L=\mathbf{13 0} ^{\circ}$ $(1+2=3 pts)$

a) Déterminer deux angles adjacents et opposés
b) Calculer la mesure de $F \widehat{O}G$ et $O \widehat{G} K$ en justifiant
1) Compléter par le signe ” < ” ou ” >” :: $(1,5 pts)$
$+2,67 < 5,01$
$ -70,7 <- 1,5$
$+13 >- 23$
2) Ranger les nombres suivants dans l’ordre croissant: : $(1 pt)$
$-19<-11,01<-9,9<-7,12<0<1<+3,1 <11$
3) On Considère la droite graduée ci-dessous : $(1+1=2 pt)$
a.
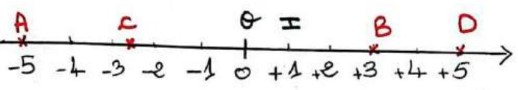
b. Calculer les distances $AB$ et $CD$ .
La distance $AB$ :
(Abscisse $_{B}-$ Abscisse $\left._{A}\right)=(3-(-5))=8$
La distance $C D$ :
(Abscisse $_{D}-$ Abscisse $\left._{C}\right)=(+5-(-2,5))=7,5$
4) Calculer les expressions suivantes en écrivant les étapes intermédiaires : $(0,5+0,5+0,5+0,5+2=4pts)$
$(-3)+7=+(7-3)=4 $
$-90 \div 45=-2$
$(+2)-(-18) =2+18=20$
$ (-18) \times(+0,5)=-9 $
$(-6)+(-6) \times(+2)+(-36) \div(-3)+3 \times(+2) =(-6)+(-12)+12+6 =(-18)+18=0$
5) Sachant que $a+b=-3$ et $a \times b=4$, calculer : $(0,75+0,75 =1,5 pt)$
• $ 1+a+(-5)+b+(-7) $
$ =a+b+1+(-5)+(-7) $
$ =3+1+(-1 2) $
$ =4+(-1 2)=-8 $
• $ a \times(-1) \times b-(-4) $
$ =a \times b \times(-1)+4 $
$ =4 \times(-1)+4 $
$ =-4+4=0 $
6) Supprimer les parenthèses puis effectuer les calculs : $(1 pt)$
$ D=-(-1 2-(+3-7))+(-12+4) $
$ D=-(-1 2-3+7)-1 2+4 $
$ D=+12+3-7-12+4 $
$ D=+12-1 / 2+4+3-7 $
$ D=+7-7 $
$D=0$
1er cas:
$A B=7 \mathrm{~cm}, A \hat{B C}=95^{\circ} ; B \hat{A C}=8$
Puisque: $A \hat{B } C+B \hat{A} C=95^{\circ}+87^{\circ}=182^{\circ}$
Donc on ne peut pas construire ce triangle, car la somme des mesures des trois angles d’un triangle est égale a $180^{\circ}$
2ème cas :
$A B=3 \mathrm{dm}=30 \mathrm{~cm} ; A C=4 \mathrm{~cm} ; B C=6 \mathrm{~cm}$
On a : $A B>A C+B C$
Donc : on ne peut pas construire ce triangle.
3ème cas :
$A B=5 \mathrm{~cm} ; A C=5 \mathrm{~cm} ; B \hat{A} C=60^{\circ} ; A \widehat{B} C=90^{\circ}$
On a $A B C$ un triangle rectangle en $B$ car $\cos A \hat{B }C=90^{\circ}$
Et aussi le triangle isocèle car: $A B=A C=5 \mathrm{~cm}$
Alors : Il faut que les mesures de ses angles à la base sont égaux à $45^{\circ}$ et non à $B \hat{A }C=60^{\circ}$
Donc : c’est impossible de construire ce triangle.
1)
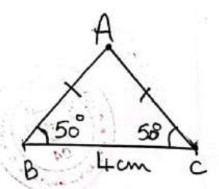
2) On sait que $A B C$ est un triangle isocèle
Alors: $\hat{A B C}=\hat{A C B}=50^{\circ}$
Et on sait que la somme des mesures des angles d’un triangle est égale a $180^{\circ}$.
Donc : $180^{\circ}-\left(50+50^{\circ}\right)=180^{\circ}-100^{\circ}=80^{\circ}$
Alors: $\hat{B A C}=80^{\circ}$
3) On a : $E \widehat{O} L=90^{\circ}$ et $N \widehat{K}M=\mathbf{6 0} ^{\circ}$ et $F \widehat{O} L=\mathbf{13 0} ^{\circ}$
• Les angles adjacents: $L\hat{O}K$ et $K\hat{O}G$
• Les angles opposés: $K\hat{O}G$ et $E\hat{O}L$
4)
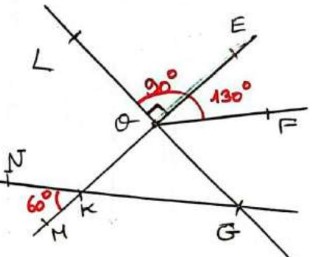
• $O\hat{G}K ?$
On a $N \hat{K} M$ et $O \hat{K} G$ deux angles apposés
Alors: $N \hat{K} M=O \hat{K} G=60^{\circ}$
Et on a: $L \hat{O} E=K \hat{O} G$ (deux angles apposés)
Alors: $K \hat{O} G=90^{\circ}$.
• Dans le triangle $O G K$ :
$O \hat{G} K=180^{\circ}-\left(90^{\circ}+60^{\circ}\right)= 180^{\circ}-150^{\circ}$
Donc : $O \hat{G} K=30^{\circ}$
• $F\hat{O}G ?$
On a: $G \hat{O} L$ un angle plat (180 )
Alors: $F\hat{O}G=G \hat{O} L-F \hat{O} L$
$F\hat{O}G=180^{\circ}-130^{\circ}$
Donc : $\hat{F O G}=50^{\circ}$
Modèle N°4
Exercice 1:$(4,25 pts)$
$1)$ Placer sur une droite graduce les paints ci-apres enchoisissand bien l’échelle de graduation: $(0,25 \times 9=2,25 pts)$
$2)$ Ranger les abscisses de ses points par ordre croissant : $(2 pts)$
Exercice 2:$(3pts)$
Recopier at compléter par deux extiers relatifs consécutifs :
$ a) \ldots<-5,4<\ldots $
$ b) \ldots<-9,4<\ldots $
$ c) \cdots<19,51<\cdots$
$d) \cdots<-1,4<\ldots .$
$e) \cdots<29,4<\ldots .$
$f) \cdots<-8,1<\ldots .$
Exercice 3:$(1 \times 6=6 pts)$
Effectuer les calculs suivants :
$(+9)+(+36)$
$(-4,5)+(-9,4)$
$(-6)+(-17) $
$(-65)-(-10)$
$ (+10)+(-5)+(-16)$
$(+17)+(+13)+(-45)$
Exercice 4:$(0,25+0,5+1,5+1,5+3=6,75pts)$
$1)$ Tracer une droite $(D)$
$2)$ Placer deux points $A$ et $B$ tels que: $A$ et $B$ ne sont pas sur la droite $(D)$
$3)$ Tracer la parallèle ( $D^{\prime}$ ) à la droite ( $D$ ) passant par $A$
$4)$ Tracer la droite ( $D^{\prime\prime}$ ) passant par $B$ et perpendiculaire à la droite $(D)$
$5)v Que peut-on dire des droites ( $D^{\prime}$ ) et ( $D^{\prime\prime}$ ) ? Justifier
$1)$
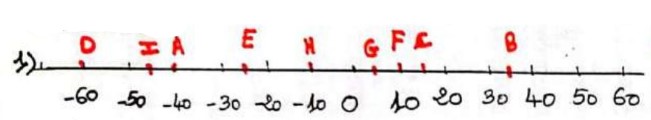
$2)$ $ -60<-45<-40<-25<-10<5<10<15<35$
$a) -6<-5,4<-5$
$b) -80<-9,4<-9$
$c)19<19,51<20$
$d) -2<-1,4<-1$
$e) 29<29,4<30$
$f) -9<-8, 1<-8$
$(+9)+(+36)=+(9+36)=+45 $
$(-4,5)+(-9,4)=-(4,5+9,4) =-13,9$
$(-6)+(-17) =-(6+17) =-23$
$(-65)-(-10) =(-65)+(+10) =-(65-10) =-55 $
$(+10)+(-5)+(-16)=(+10)+(-21) =-(21-10) =-18 $
$(+17)+(+13)+(-45)=(+30)+(-45)=-(45-30) =-15$

$5)$ On sait que:
$( D^{\prime} )$ // $( D )$
$( D^{\prime\prime} )$ ⊥ $( D )$
Or, si deux droites sont parallèles, alors toute droite perpendiculaire à l’une est perpendiculaire à l’autre.
Donc :$\left(D^{\prime \prime}\right) \perp\left(D^{\prime}\right)$
Devoirs Corrigés Maths N°2 S1 1AC