Devoirs Corrigés Maths N°3 S1 3AC
Modèle N°1
Exercice 1:$(4×0,5=2$ pts)
$1)$ Compléter par: Vrai ou Faux
* Dans un triangle rectangle, l’hypoténuse est le plus grand côté$……..$
* Le sinus d’un angle aigu est égal au quotient du côté adjacent sur l’hypoténuse$……..$
* Si $\hat{x}$ un angle aigu, alors : $\cos {\hat{x}} +\sin {\hat{x}} =1$$……..$
* Si un angle inscrit et un angle au centre interceptent le même arc, alors ils ont la même mesure$……..$
Exercice 2:$(1,5+1,5+1,5+2+1,5=8$ pts)
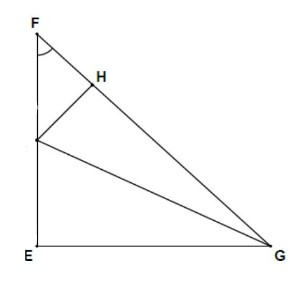
$1)$ Soit $E F G$ un triangle, avec : $E F=4 ; E G=2 \sqrt{5}$ et $F G=6$.
• Montrer que $E F G$ est un triangle rectangle et préciser en quel sommet.
$2)$ Calculer $: \boldsymbol{\operatorname { c o s }} \widehat{\boldsymbol{F}}; \boldsymbol{\operatorname { s i n }} \widehat{\boldsymbol{F}} ; \boldsymbol{\operatorname { t a n }} \widehat{\boldsymbol{F}}$
$3)$ Calculer $: \boldsymbol{\operatorname { c o s }} \widehat{\boldsymbol{G}} ; \sin \widehat{\boldsymbol{G}} ; \tan \widehat{\boldsymbol{G}}$
$4)$ Soit $I$ le milieu de $[E F]$ et $H$ sa projeté orthogonal sur la droite (FG).
$4-1) $ Calculer $I G$.
$4-2)$ Calculer $I H$.
Exercice 3:$(1 + 1+ 1,5+1,5+2=7$ pts)
Soit un triangle $EDF$ rectangle en $D$.
$1)$ Soit $x$ un angle aigu tel que : $\cos x=\frac{2}{5}$.
$1-1)$ Calculer: $\sin x$
$1-2)$ Calculer: $\tan x$
$2)$ Calculer :
$A=2 \cos ^{2} 17^{\circ}+1+2 \sin ^{2} 17^{\circ}$
$B=3 \sin ^{2} 10^{\circ}+\sqrt{5} \cos 20^{\circ}+3 \sin ^{2} 80^{\circ}-\sqrt{5} \sin 70^{\circ}-\tan 50^{\circ} \times \tan 40^{\circ}$
$3)$ Montrer que : $\frac{(\cos x+\sin x)^{2}-1}{1-\cos ^{2} x}=\frac{2}{\tan x}$
Exercice 4:$(1 + 1+ 1 = 3$ pts)
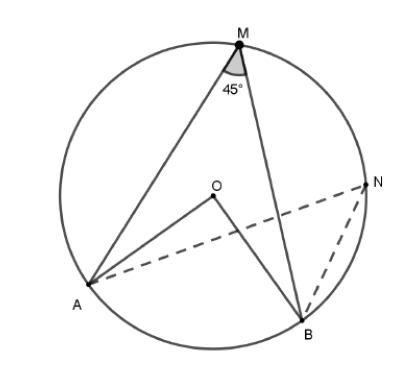
Soit la figure ci-contre tel que $O$ est le centre du cercle.
$A, B, N$ et $M$ sont des points du cercle tel que : $\widehat{A M B}=45^{\circ}$.
$1)$ Déterminer la mesure de l’angle $\widehat{A N B}$. Justifier votre réponse.
$2)$ Déterminer la mesure de l’angle $\widehat{A O B}$. Justifier votre réponse.
$3)$ Déduire que le triangle $A O B$ est rectangle isocèle.
$1)$ Compléter par: Vrai ou Faux
* Vrai
* Faux
* Faux
* Faux
$1)$ Soit $E F G$ un triangle, avec : $E F=4 ; E G=2 \sqrt{5}$ et $F G=6$.
$F G^{2}=6^{2}=36$ et $E F^{2}+E G^{2}=4^{2}+(2\sqrt{5})^{2}=16+20=36$
Puisque : $E F^{2}+E G^{2}=F G^{2}$
Alors d’après la réciproque du théorème de Pythagore le triangle $ EFG$ est rectangle en $ E$.
$2)$ Calculer :
$ \boldsymbol{\operatorname { c o s }} \widehat{\boldsymbol{F}}=\frac{EF}{FG}=\frac{4}{6}=\frac{2}{3}$
$ \boldsymbol{\operatorname { s i n }} \widehat{\boldsymbol{F}}=\frac{EG}{FG}=\frac{2\sqrt{5}}{6}=\frac{\sqrt{5}}{3}$
$\boldsymbol{\operatorname { t a n }} \widehat{\boldsymbol{F}}=\frac{EG}{EF}=\frac{2\sqrt{5}}{4}=\frac{\sqrt{5}}{2}$
$3)$ Calculer :
$ \boldsymbol{\operatorname { c o s }} \widehat{\boldsymbol{G}} =\frac{EG}{FG}=\frac{2\sqrt{5}}{6}=\frac{\sqrt{5}}{3}$
$ \sin \widehat{\boldsymbol{G}}=\frac{EF}{FG}=\frac{4}{6}=\frac{2}{3}$
$ \tan \widehat{\boldsymbol{G}}=\frac{EF}{EG}=\frac{4}{2\sqrt{5}}=\frac{2}{\sqrt{5}}$
$4)$ Soit $I$ le milieu de $[E F]$ et $H$ sa projeté orthogonal sur la droite (FG).
$4-1) $ Calculer $I G$.
Le triangle $IEG$ est rectangle en $E$.
Alors d’après le théorème direct de pythagore :
$I G^{2}= IE^{2}+E G^{2}$
$I G^{2}= 2^{2}+(2\sqrt{5})^{2}$
$I G^{2}= 4+20$
$I G^{2}=24$
$I G=2\sqrt{6}$
$4-2)$ Calculer $I H$.
On a : $ \sin \widehat{E\boldsymbol{F}G}=\sin \widehat{I\boldsymbol{F}H}$
C’est à dire que : $\frac{EG}{FG}=\frac{IH}{FI}$
Donc : $\frac{2\sqrt{5}}{6}=\frac{IH}{2}$
Alors : $IH=\frac{2\sqrt{5}}{6}×2$
Donc : $IH=\frac{2\sqrt{5}}{3}$
$1)$ Soit $x$ un angle aigu tel que : $\cos x=\frac{2}{5}$.
$1-1)$ Calculons $\sin x$ :
On sait que : $\cos ^{2}(x)+\sin ^{2}(x)=1$
Alors : $\sin ^{2}(x)=1-\cos ^{2}(x)=1-\left(\frac{2}{5}\right)^{2}=1-\frac{4}{25}=\frac{21}{25}$
Donc : $\sin x=\sqrt{\frac{21}{25}}=\frac{\sqrt{21}}{5}$
$1-2)$ Calculons $\boldsymbol{\operatorname { t a n }} \boldsymbol{x}$ :
On sait que $: \tan x=\frac{\sin x}{\cos x}=\frac{\frac{\sqrt{21}}{5}}{\frac{2}{5}}=\frac{\sqrt{21}}{2}$
$2)$ Calculer :
$A=2 \cos ^{2} 17^{\circ}+1+2 \sin ^{2} 17^{\circ}$
$A=2 \cos ^{2} 17^{\circ}+2 \sin ^{2} 17^{\circ}+1$
$A=2×( \cos ^{2} 17^{\circ}+\sin ^{2} 17^{\circ})+1$
$A=2×( 1)+1$
$A=3$
$B=3 \sin ^{2} 10^{\circ}+\sqrt{5} \cos 20^{\circ}+3 \sin ^{2} 80^{\circ}-\sqrt{5} \sin 70^{\circ}-\tan 50^{\circ} \times \tan 40^{\circ}$
$B=3 \sin ^{2} 10^{\circ}+3 \sin ^{2} 80^{\circ}+\sqrt{5} \cos 20^{\circ}-\sqrt{5} \sin 70^{\circ}-\tan 50^{\circ} \times \tan 40^{\circ}$
$B=3 \sin ^{2} 10^{\circ}+3 \cos ^{2} 10^{\circ}+\sqrt{5} \cos 20^{\circ}-\sqrt{5} \cos20^{\circ}-\tan 50^{\circ} \times \frac{1}{\tan 50^{\circ} }$
$B=3×1- \frac{\tan 50^{\circ}}{\tan 50^{\circ} }$
$B=3×1- 1$
$B=2$
$3)$ Montrer que : $\frac{(\cos x+\sin x)^{2}-1}{1-\cos ^{2} x}=\frac{2}{\tan x}$
$\frac{(\sin x+\cos x)^{2}-1}{1-\cos ^{2} x}=\frac{\left(\sin ^{2} x+2 \cos x \times \sin x+\cos ^{2} x\right)-1}{\sin ^{2} x}$
$\frac{\left(\sin ^{2} x+2 \cos x \times \sin x+\cos ^{2} x\right)-1}{\sin ^{2} x}=\frac{\left(\sin ^{2} x+\cos ^{2} x+2 \cos x \times \sin x\right)-1}{\sin ^{2} x}$
$\frac{(1+2 \cos x \times \sin x)-1}{\sin ^{2} x}=\frac{2 \cos x \times \sin x}{\sin ^{2} x}=\frac{2 \cos x}{\sin x}=\frac{2}{\tan x}$
$1)$ Déterminer la mesure de l’angle $\widehat{A N B}$. Justifier votre réponse.
$\widehat{A N B}$ et $\widehat{A M B}$ deux angles inscrits interceptent le même arc $\overparen{A B}$ , alors ils ont la même mesure.
$\widehat{A N B}=\widehat{A M B}=45°$
$2)$ Déterminer la mesure de l’angle $\widehat{A O B}$. Justifier votre réponse.
On a $\widehat{A O B}$ est un angle au centre et $\widehat{A M B}$ est un angle inscrit interceptent le même arc $\overparen{A B}$.
Donc : $\widehat{A O B}=2×\widehat{A M B}=2×45°=90°$
$3)$ Déduire que le triangle $A O B$ est rectangle isocèle.
On a : $\widehat{A O B}=90°$
Et : $OA=OB$ car c’est le rayon.
Donc : le triangle $A O B$ est rectangle isocèle.
Modèle N°2
Exercice 1:$(4×1,5=6$ pts)
Soit $E F G$ un triangle tel que: $E F=5 ; E G=1 2$ et $F G=13$ .
• Calculer $\cos \hat{F}$ , $\tan \hat{F}, \sin \hat{F} \quad et \quad \sin \hat{G}$ .
Exercice 2:$(2 + 2+ 2+1,5+1,5=9$ pts )
$1)$ Soit $x$ un angle aigu tel que :$ \frac{4 \cos x-9 \sin x}{2 \cos x-3 \sin x}=4 $
• Calculer $\tan x$
$2)$ Soit $x$ un angle aigu tel que : $\cos x-\sin x=\sqrt{2} \cdot \sin x$
$a)$ Montrer que: $\cos x+\sin x=\sqrt{2} \cos x$
$b)$ Calculer $\cos x$ et $\sin x$
$3)$ Calculer
$ A=3 \cos 37^{\circ}+\sin ^{2} 19^{\circ}+\sin ^{2} 71^{\circ}+3 \cos ^{2} 53^{\circ} $
$ B=\frac{1}{\tan ^{2} 57^{\circ}+1}-\frac{\tan ^{\circ} 33^{\circ}}{\tan ^{2} 33^{\circ}+1}$
Exercice 3:$(1,5+2+1,5=5$ pts )
Soit la figure ci-contre tel que $\theta$ est le centre du cercle.
$A, B, C$ et $M$ sont des points du cercle tel que: $A B C$ est un triangle équilatéral.
$1)$ Déterminer la mesure de l’angle $A \widehat{M }B$. Justifier votre réponse.
$2)- a)$ Déterminer la mesure de l’angle $A \widehat{M }C$, puis déduire la mesure de l’angle $B \widehat{M }C$.
$b)$ Déterminer la mesure de l’angle $\hat{A O B}$
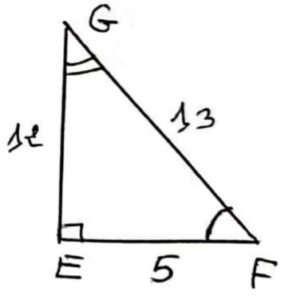
On doit montrer que $EFG$ est un triangle rectangle.
On a : $E F^{2}+E G^{2}=5^{2}+1 2^{2}=25+144$
$E F^{2}+E G^{2}=169$
et $F G^{2}=133^{2}=169$
Alors: $E F^{2}+E G^{2}=F G^{2}$
Alors d’après la réciproque du théorème de Thalès, le triangle $E F G$ est rectangle en $E$
$ \tan \hat{F}=\frac{E G}{E F}=\frac{1 2}{5} $
$ \sin \hat{G}=\frac{E F}{F G}=\frac{5}{13} \$
$\sin \hat{F}=\frac{E G}{F G}=\frac{12}{13} $
$ \cos \hat{F}=\frac{E F}{F G}=\frac{5}{13}$
$1)$ Calculons $\tan x$ :
On a: $ \frac{4 \cos x-9 \sin x}{2 \cos x-3 \sin x}=4$
Alors: $4(2 \cos x-3 \sin x)=4 \cos x-9 \sin x$
$8 \cos x-12 \sin x =4 \cos x-9 \sin x $
$8 \cos x-4 \cos x =-9 \sin x+12 \sin x $
$4 \cos x =3 \sin x$
Donc: $\quad \frac{\sin x}{\cos x}=\frac{4}{3}$
D’où :
$\tan x=\frac{4}{3}$
$2)$ $a)$ Montrons que: $\cos x+\sin x=\sqrt{2} \cos x$
on a: $\cos x-\sin x=\sqrt{2} \sin x$
Alars: $\quad \cos x=\sqrt{e} \sin x+\sin x$
c.a.d $\cos x=(\sqrt{2}+1) \sin x$
on a : $\cos x+\sin x=\sqrt{2} \sin x+\sin x+\sin x$
$\cos x+\sin x=\sqrt{2} \sin x+2 \sin x $
$\cos x+\sin x=\sqrt{2} \sin x \cdot(\sqrt{2}+1)$
$\cos \sqrt{2} \times \sqrt{2}=2$
Alors: $\cos x+\sin x=\sqrt{2} \cos x$
Donc: $\Rightarrow \cos x+\sin x=\sqrt{2} \cos x$
$b)$ Calculons $\cos x$ et $\sin x$ :
On a: $\cos x+\sin x=\sqrt{2} \cos x$
C.à.d. $\quad \sin x=\sqrt{1} \cos x-\cos x$
$\sin x=(\sqrt{2}-1) \cos x$
on sait que: $\sin ^{2} x+\cos ^{2} x=1$
Alors: $\cos ^{2} x+[(n-1)+x]^{2}=1$
$ \cos ^{2} x+(\sqrt{2}-1)^{2} \cos ^{2} x=1 $
$ \cos ^{2} x\left(1+(\sqrt{2}-1)^{2}\right)=1$
$\cos ^{2} x(1+(2-2 \sqrt{2}+1))=1 $
$\cos ^{2} x(1+3-2 \sqrt{2})=1 $
$\cos ^{2} x(4-2 \sqrt{2})=1$
$\cos ^{2} x(4-2 \sqrt{2})=1 $
$2(2-\sqrt{2}) \cos ^{2} x=1 $
$\cos ^{2} x=\frac{1}{2(2-\sqrt{2})} $
$\cos ^{2} x=\frac{2+\sqrt{2}}{2(2-\sqrt{2})(2+\sqrt{2})}$
C.à.d. $\cos ^{2} x=\frac{2+\sqrt{2}}{2\left(2^{2}-\sqrt{2}^{2}\right)}=\frac{2+\sqrt{2}}{2(4-2)} $
$ \cos ^{2} x=\frac{2+\sqrt{2}}{4}$
Alors : $\cos x=\frac{\sqrt{2+\sqrt{2}}}{2}$
On sait que: $\sin ^{2} x+\cos ^{2} x=1$
D’où: $\cos ^{2}: \sin ^{2} x =1-\cos ^{2} x $
$\sin ^{2} x =1-\left(\frac{2+\sqrt{2}}{4}\right)$
Alors: $\sin ^{2} x=\frac{4-(2+\sqrt{2})}{4}$
$\sin ^{2} x=\frac{2-\sqrt{2}}{4}$
D’où: $\cos : \sin x=\frac{\sqrt{2-\sqrt{2}}}{2}$
$3)$ $A=3 \cos ^{2} 37^{\circ}+\sin ^{2} 19^{\circ}+\sin ^{2} 71^{\circ}+3 \cos ^{2} 53^{\circ}$
On a: $37^{\circ}+53^{\circ}=90^{\circ}$ et $19^{\circ}+71^{\circ}=90^{\circ}$
Alors :
$A =3 \cos ^{2} 37^{\circ}+\sin ^{2} 19^{\circ}+\sin ^{2} 71^{\circ}+3 \cos ^{2} 53^{\circ} $
$A =3 \cos ^{2} 37^{\circ}+\sin ^{2} 19+\cos ^{2} 19^{\circ}+3 \sin ^{2} 37^{\circ}$
$A =3\left(\cos ^{2} 37^{\circ}+\sin ^{2} 37\right)+\left(\sin ^{2} 19^{\circ}+\cos ^{2} 19^{\circ}\right) $
$A =3 \times 1+7 $
$A =4$
$ B=\frac{1}{\tan ^{2} 57^{\circ}+1}-\frac{\tan ^{2} 33}{\tan ^{2} 33^{\circ}+1}$
On a: $57^{\circ}+33^{\circ}=90^{\circ}$
D’où: $\tan 57^{\circ}=\frac{1}{\tan 33^{\circ}}$
Alors: $\frac{\beta}{\tan ^{2} 57^{\circ}+1}=\frac{\beta}{\frac{\beta}{\tan 33^{\circ}}+1}$
$B=\frac{\beta}{\frac{s+\tan 33^{\circ}}{\tan 33^{\circ}}}=\frac{\tan 33^{\circ}}{1+\tan 33^{\circ}} $
$B=0$
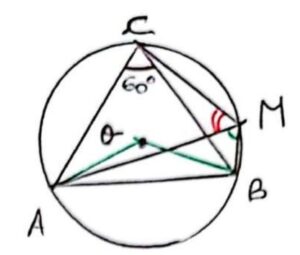
$1)$ $A \hat{M} B$ ?
On a $A B C$ un triangle équilatéral.
Alors :
$A \hat{B} C =C \hat{A}B=B \hat{A} C=60^{\circ}$
Et on sait que l’angle inscrit $\hat{A M B}$ et l’angle inscrit $A \hat{C} B$ interceptent le même arc $\widehat{A B}$, alors ses angles ont même mesure.
Donc: $\hat{A M B}=\hat{A C B}=60^{\circ}$
Alors: $A \hat{M B}=60^{\circ}$
$2)$$a)$ $A \hat{M} C$?
On a les deux angles inscrits $A \hat{M} C$ et $A \hat{B }C$ interceptent le même arc, alors ces angles ont même mesure.
D’où : $A \hat{M} C=A \hat{B} C=60^{\circ}$
Alors $A \hat{M} C=60^{\circ}$
(*) $B \hat{M} C$
On a: $\quad B \hat{M} C=A \hat{M} B+A \hat{M} C$
Alors: $B \hat{M} C=120^{\circ}$
$b)$ On sait que l’angle inscrit $A \widehat{C B}$ intercepte le même arc $\widehat{A B}$ que l’angle au centre $\hat{A O B}$.
Donc: $A \hat{O} B=2 A \hat{C} B$
Or: $A \hat{C} B=60^{\circ}$
Alors: $A \hat{O} B=2 \times 60=120^{\circ}$
Devoirs Corrigés Maths N°3 S1 3AC